Basic Maths for Protein Crystallographers
	Phasing diagrams

SIR - Single Isomorphous Replacement

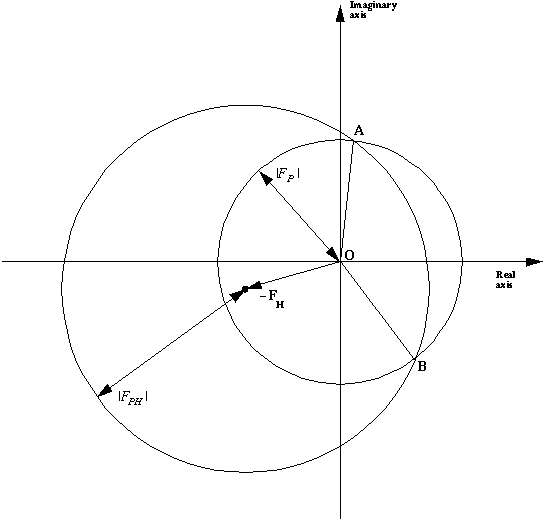
Phasing diagram for Single Isomorphous Replacement.

Two circles are drawn, one with radius |F_P| centred on the origin, and one with radius |F_PH| about the end of the vector -F_H. The two points of intersection A and B of the two circles correspond to two possible values of the protein phase. The mean or "best" protein phase for such a circle will always equal (h) or (h)+. The "figure of merit" is a measure of the phase error. Here it equals cos_diff and indicates how separate the two crossings are. If _diff = 90 then the FOM will equal 0.0.

If the heavy atom constellation is on the wrong hand, the resultant phase estimates for _P will also be on the wrong hand, but the figure of merits will be identical.

The phase ambiguities are:
Crossing	Hand
(h)1 + diff1 (h)1 - diff1	-(h)1 + diff1 -(h)1 - diff1
best = (h)1 or (h)1+	best = -(h)1 or -(h)1+

Maps from different hands will be enantiomorphic.

MIR - Multiple Isomorphous Replacement

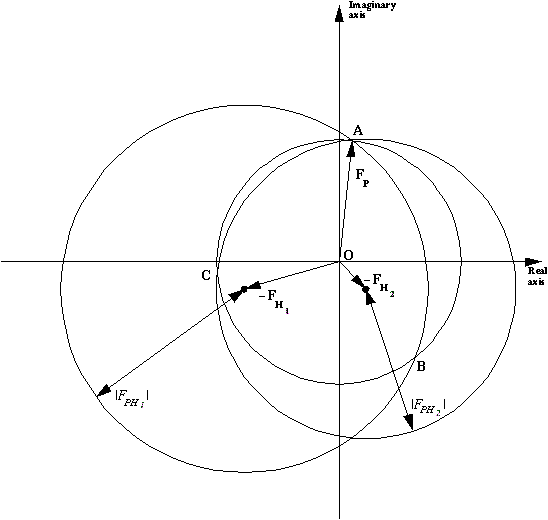
Phasing diagram for Multiple Isomorphous Replacement.

When two derivatives are available, both with heavy atoms positioned on the same origin and hand, a third circle can be drawn, and in favourable circumstances the three circles intersect in one clearly defined point (point A in the diagram), thus resolving the phase ambiguity.

To make sure the two derivatives have heavy atoms on the same origin and same hand, it is important to use difference Fouriers to position the second derivative, using phases for the protein determined from the first derivative.

In this case no anomalous measurements for the derivatives are available, and if the heavy atoms structure factor is calculated on the other hand, a mirror image of the figure across the (h)=0 line will be generated, changing all phases to their complex conjugates, including the resultant phase estimate for the protein. The resultant map will be the enantiomorph of the first one, so both should show clear solvent boundaries, and look "good", but one will show features like left-handed helices.

The phase ambiguities are:
Crossing	Hand
(h)1 + diff1 (h)1 - diff1	-(h)1 + diff1 -(h)1 - diff1
(h)2 + diff2 (h)2 - diff2	-(h)2 + diff2 -(h)2 - diff2

In this figure there is a good crossing at (₂-_diff2, ₁+_diff1).
And in the corresponding one for the other hand, this would be at (-₂+_diff2, -₁-_diff1).

SIRAS - Single Isomorphous Replacement with Anomalous Scattering

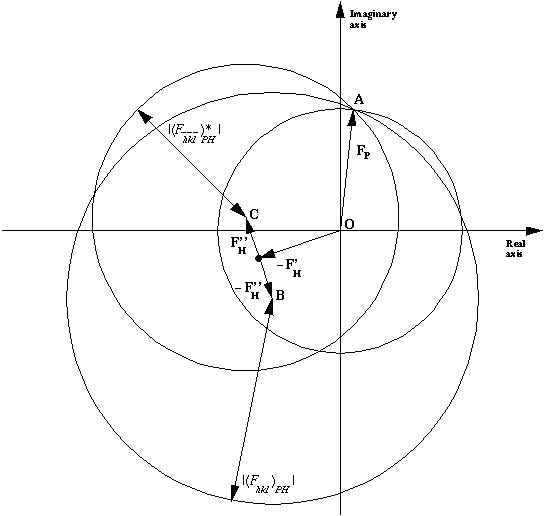
Phasing diagram for SIR with Anomalous Dispersion.

Referring to the SIR phase, the F_P(h) phase will be _H(h) + _diff(h) or _H(h) - _diff(h). And for F_P(-h) the phase will be _H(-h) + _diff(-h) or _H(-h) - _diff(-h). The anomalous scattering of the heavy atoms means that _H(-h) is not the complex conjugate of _H(h). And since |F_PH(+h)| is not equal to |F_PH(-h)| the _diff(h) is also different from _diff(-h). However the phase of F_P(-h) should equal -phase of F_P(+h), so we can select which of the crossing is the most likely. To represent this using phasing circles we actually use a trick.

We consider F_PH(-h) as another "derivative" F'_PH(h) where the heavy atom contribution equals F_Hreal(h)e^i((h)) + F"_Himag(h) e^{i((h) - 90)} with the anomalous contribution lagging 90 degrees BEHIND the real contribution.
This means that like in the MIR case, there are two centres for F_Hi, but of course they are always rather close. Taking F_PH(h) as F_PH1(h) and F_PH(-h) as F_PH2(h), we can position the centres of the two "F_H" vectors correctly, and in this figure the resultant _P will equal (h)₁+_diff1, or (-h)₁-_diff1. If the heavy atoms are on the other hand, (h)₂ and (-h)₂ are no longer the complex conjugates of (h)₁ and (-h)₁ so although the crossings will be equally reliable at ((h)₂+ _diff1, (-h)₂- _diff1) and the figures of merit will be identical, this time the protein phase will not be the enantiomorph.
Hence maps using the 2 different phases are not equivalent, and you should be able to recognise that one is "better" than the other, and indicates the correct hand.

The phase ambiguities are:
Crossing	Hand
(h)1+ + diff+ (h)1+ - diff+	(h)2+ + diff+ (h)2+ - diff+
(h)1- + diff- (h)1- - diff-	(h)2- + diff- (h)2- - diff-

Rewording this, the effect of anomalous scattering is to introduce a phase shift, which is different for reflections forming a Friedel pair. Thus there are circles with radii |(F_hkl)_PH| and |(F_-h-k-l)^*_PH| centred at -(F_H+F"_H) and -(F_H-F"_H) (points B and C in the diagram), intersecting with the circle corresponding to |F_P| in only one point (point A in the diagram).

MIRAS - Multiple Isomorphous Replacement with Anomalous Scattering

No picture available yet

This can be seen as a merging of the MIR and the SIRAS cases. There should now be no phase ambiguity, and in fact refining the anomalous "occupancy" starting from Aocc = 0.0 should result in these occupancies all becoming positive, indicating the hand is correct, or all negative indicating it is wrong.

SAD - Single Anomalous Dispersion

For picture see SIRAS case

The SIRAS figure can be used to illustrate the case of SAD phasing assuming F_Hreal is zero. Here, as in the SIR phasing, again there are only two measurements to consider: F_PH(h) and F_PH(-h). The crossings will now indicate a phase of _H+90±_diff, and as in the SIR case the "best" phases will be (h)+90, and the figure of merit equals cos_diff. If the hand of the heavy atoms is changed, the phase will change to -_H+90±_diff, the "best" phase will be -(h)+90, and the figure of merit will be the same. In this case the map on the other hand is actually a Babinet or negated copy of the inverse of the true map, i.e. _best2 = -_best1 + 180.

The phase ambiguities are:
Crossing	Hand
(h)1 + 90 + diff1 (h)1 - 90 + diff1	-(h)1 + 90 + diff1 -(h)1 - 90 + diff1
best = (h)1 + 90 or (h)1 + 90 +	best = -(h)1 + 90 or -(h)1 + 90 +

Maps from different hands will be enantiomorphic Babinet maps, i.e. all negative density in one becomes positive density in the other, since the _best(-) = -_best(+) + 180.